The dynamical system in simple words is really a system whose condition changes with time. The instance includes the mathematical model that describes population growth, swinging of the pendulum, or predator-prey model. There’s two kinds of dynamical systems: iterated maps and differentials equations. The iterated maps are utilized to solve problems where time is discrete, whereas the differential equation can be used to resolve problems where time is continuous.
Now confining our focus on differential equations, it may be split into various kinds: Ordinary or Partial and Straight line or Nonlinear, etc. Within this report, we’ll cope with Ordinary differential equations and Nonlinear differential equations.
We are able to understand the thought of an engaged system, ordinary and nonlinear differential equations by utilizing one particualr population growth model. In almost any population, the amount of individuals changes with time. The modification in population can happen because of various reasons, for instance, resource availability, competition, and disease. The easiest model describing alterations in the populace dimensions are the exponential growth model. For simplicity, we’ll think that the populace doesn’t communicate with its atmosphere and doesn’t get impacted by ecological changes. And so the equation is going to be
dn(t)/ dt = rn
Where dn(t)/ dt is really a first degree ordinary differential equation, where r is really a rate of growth and n within the population size. Figure 1, shows an exponential development in population.
Figure 1: Exponential development in population.
Synchronization — the operation or activity of several things simultaneously or rate, frequently happens in nature. For instance, neurons within our brain, cardiac pacemaker cells, blinking of fireflies in symphony.
Oscillators really are a system that has some type of periodic movement and shows steady condition conduct during a period of time [1]. Anyway some systems become synchronized with the oscillation phenomena. Oscillator is a big section of mathematics, therefore, we’ll only describe phase oscillators within this report.
Entertainment of fireflies
Fireflies would be the soft bodied beetles generally referred to as lightning bugs for his or her utilization of bioluminescence during twilight to draw in mates. Once the fireflies start to imitate light. The fireflies blink in their own pace and frequency, however there comes a period when a large number of fireflies start flashing off and on all at one time. The majority of the fireflies can adjust their natural frequencies to match using the fireflies around it.Here, every firefly is attempting to dynamically synchronize its frequency with this of each and every other firefly. This synchronization phenomenon continues to be studied by many people scholars. We’re going for a simplified model in the chapter on Firefly in the book “;Nonlinear Dynamics and Chaos” -by Steven H. Strogatz. Within the model
Within this chapter, Strogatz examines one where firefly reacts to rhythm of the flashing stimuli. Let’s think that the phase from the firefly’s flashing is offered by θ(t) where θ= may be the instant once the firefly emits the flash. We think that θ is 2π-periodic. Therefore, θ= 2πn. Then it’s natural frequency -the regularity where it blinks without worrying about a stimuli be θ=ω. Similarly, now let’s introduce a stimulus with 2π-periodic phase Θ(t) where Θ= may be the instant from the stimulus flashing, and Θ = Ω is its frequency.
As you may know that, firefly will endeavour to synchronize using the stimulus, when the stimulus flashes following the firefly then firefly will endeavour to slow. Similarly, when the stimulus is flashing prior to the firefly then your firefly will endeavour to hurry as much as synchronize using the stimulus. We are able to describe this model by having an equation as
Θ =˙ ω + A crime(Θ − θ) (1)
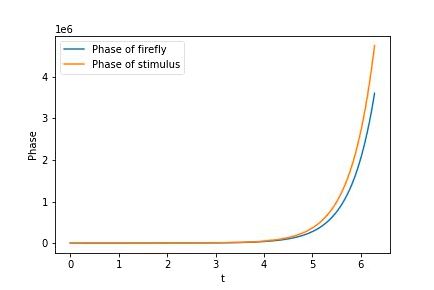
In Which A is really a way of measuring ale firefly to alter its frequency as a result of a stimulus, and (Θ − θ) is really a phase distinction between the stimulus and also the firefly. To be able to possess a better picture of the above equation (1), we plotted a graph by providing a initial value for ω , Ω along with a. Figure 2, shows the bend for phases of firefly and also the stimulus.
Figure 2: Phase of firefly and stimulus.
Out of this equation we are able to imply if < Θ − θ < π, meaning stimulus is in front of the firefly, meaningΘ˙ > ω, and so the firefly accelerates. Similarly, if −π < Θ − θ < , meaning Θ˙ < ω, and also the firefly slows lower.
Now we have one, we evaluate the firefly’s reaction to the stimulus. Once the entertainment occurs, the main difference in phase between your stimulus and also the firefly φ approaches a set constant. This could show various conduct within the system : phase synchronization, phase locked, phase drift. Using equation,
φ˙= Ω − ω − A crime(φ) (2)
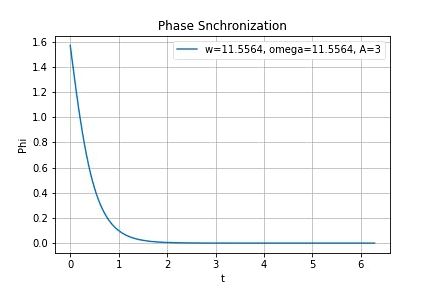
When the constant is , this means that the firefly has synchronized using the stimulus and also the firefly and stimulus are flashing together. We are able to show this phenomenon by solving equation (2) and plotting the graph for φ versus time, where time is incorporated in the selection of to 2π splitted into 1000, and also the initial value for ω and Ω is 11.5564 Hz. Figure 3 shows the phase synchronization.
Figure 3: Phase Synchronization
Similarly, when the constant isn’t it can display two different behaviours. The firefly may either maintain a phase locked towards the stimulus, and therefore firefly and also the stimulus have a similar immediate frequency, however the firefly will invariably flash behind or ahead with a fixed amount. Figure 4 shows Phase locked conduct.
Figure 4: Phase locked.
Finally, when the constant isn’t and when the regularity from the stimulus is simply too high or lacking then firefly will find it difficult to match the regularity from the stimulus, and therefore entertainment won’t occur, this conduct is called phase drift. Figure 5 shows the phase drift conduct.
Figure 5: Phase drift
Fixed Point and Stability Analysis
In the past figures (3,4,5) we had the phase difference trajectories of various conduct within the system. Now, to evaluate the soundness of those behaviours, we are able to check out the stability from the fixed points. Fixed points are based on f(x)=, it’s a point which remains stagnant once the system changes combined with the time. We are able to check out the stability by plotting a graph for φ˙ versus φ using equation (2). For every kind of conduct, there are various kinds of stability from the fixed points. For figure 6, stable fixed point reaches φ = which matches phase synchronization, for figure 7,stable fixed point reaches φ = c where c is indeed a constant, which matches phase locked, and lastly in figure 8 there aren’t any stable fixed points.
Figure 6: Phase Synchronization Figure 7: Phase Locked
Figure 8: Phase Drift
To ensure that entertainment to happen , φ should move together with c as time move combined with the infinity, here c could be any real number including , therefore, for that simplicity we consider c=, meaning fixed point , φ˙ = . From equation (2), we are able to write,
= Ω − ω − A crime(φ) (3)
crime(φ) = Ω − ω /A (4)
We all know that −1 ≤ crime(φ) ≤ 1, therefore equation 4 is going to be,
−1 ≤ Ω − ω/ A ≤ 1
−A ≤ Ω − ω ≤ A
ω − A ≤ Ω ≤ ω + A (5)
Therefore, from equation (5) we are able to state that for that entertainment to happen omega ought to be between ω − A and ω + A .
We learned that parameter A may also affect the conduct from the system. Based on the plot, bigger values of the cause phase synchronization, proven in figure 9, whereas smaller sized values cause phase drift and phase locking proven in figure 10 and figure 11 correspondingly.
Figure 9: Phase Synchronization Figure 10: Phase Locked
Figure 11: Phase Drift
Firefly synchronization with two stimuli
To date, i was using a simple model which in fact had one firefly and something stimulus. We are able to refer to this as just one stimulus situation. But exactly how will the fireflies respond to the 2 different stimuli — stimulus 1 and stimulus 2 symbolized by Θ1 and Θ2 correspondingly, meaning two different frequencies? We are able to explore this situation because they build another model. The model is going to be identical to the equation (1). For the models we’ll make use of the same parameters for phase of firefly but two different phases for 2 different stimuli. Allow the frequencies of stimulus 1 Θ1= Ω1 and also the frequency of stimulus 2 Θ2=Ω1. Allow the phase distinction between simulus 1 and firefly and phase distinction between stimulus 2 and firefly be φ1 = Θ1 − θ and φ2 = Θ2 − θ correspondingly. Let’s suppose one that predicts the phase from the firefly change be
θ = ω + A crime(φ1) + A crime(φ2) (6)
We are able to call equation (6), multiple stimuli models. We all know that φ1 = Θ1 − θ and φ2 = Θ2 − θ, and then we can write equation (6) as
φ˙ 1 = Ω1 − ω − A crime(φ1) − A crime(φ2) (7)
φ˙ 2 = Ω2 − ω − A crime(φ1) − A crime(φ2) (8)
Suppose frequencies for stimulus 1 and a pair of both of them are same, then Ω1 = Ω2. Consequently φ1 = φ2. This is the situation where we won’t have various kinds of stimuli, the model will behave as just one stimulus model.
However, when the frequencies for stimulus 1 and a pair of both of them are not same, then Ω1 isn’t comparable to Ω2. Within this situation, once the firefly will start to change its frequency to complement those of stimulus 1, phase difference φ2 will decrease or increase, however phase difference φ2 may also decrease or increase combined with the φ2. Therefore, the trajectories of φ1 and φ2 is going to be uniformly growing and decreasing. Thus the firefly won’t ever synchronize using the stimuli, but rather may cause phase drift with the stimuli.
Figure 12: Firefly conduct with two stimuli
Conclusion
We attempted to know firefly synchronization in existence of the stimulus. Within the model which in fact had just one stimulus, demonstrated three different types of conduct — Phase synchronization, Phase locked and Phase drift. Phase synchronization only takes place when frequency from the firefly and also the stimulus is identical, otherwise either the firefly and stimulus as well as in phase locked condition or phase drift condition. We attempted to evaluate conduct within the alternation in the resetting strength (A) from the firefly. We learned that large resetting strength may lead to phase synchronization, however, less A may cause either phase lock or there won’t be any entertainment whatsoever. Finally we attempted to determine the conduct from the firefly when two stimuli can be found. In the model we learned that in existence of two stimuli, when the frequencies of both stimuli is same then synchronization can happen, otherwise they’re not going to synchronize.
References
[1] The Oscillator Principle of Nature- An easy Observation
[2] Runyeon, Hope. “;Firefly Synchronization.” (2006).
[3] Strogatz, S. H. Nonlinear Dynamics and Chaos, with Applications to Physics, Biology, Chemistry, and Engineering. Studying, MA: Addison-Wesley, 1994.
Resourse:https://medium.com/swlh/design-and-analysis-of-firefly-response-to-the-stimulus-2b747e55350 Key:Synchronization in Fireflies