Secretary-General Applauds World Food Programme’s Nobel Peace Prize Award, Calling Hunger ‘an Outrage’ among Plenty, in Remarks to Executive Board Conferences
Guidance for the Conduct and Reporting of Modeling and Simulation Studies in the Context of Health Technology Assessment | Effective Health Care Program
Investing in Sustainable Urban Infrastructure | School of Business | The George Washington University
Exactly what do I study? | Jen Henderson
IDC’s New Way forward for Operations Framework Calls on Companies to get Resilient Decision Makers to attain Digital Success
How can sustainable urban infrastructure advance within the next fifteen years? Wood
Research Area: HCI EECS at UC Berkeley
Overview
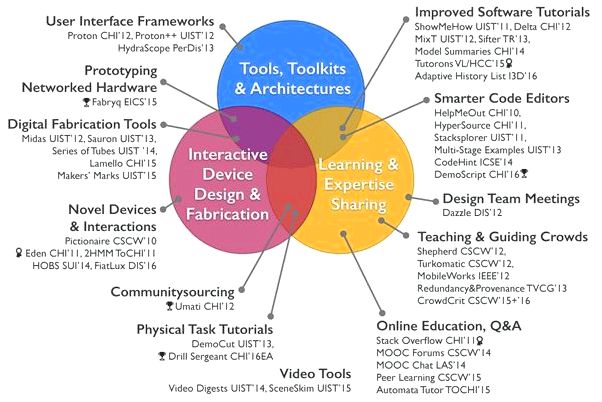
A Persons-Computer Interaction Group in EECS studies interaction in current and future computing environments, spanning workplaces, homes, public spaces, and beyond. The HCI group partcipates in collaborations with scholars and designers across campus, driving research presented at venues for example CHI, UIST, DIS, VIS, and CSCW, and helps to create novel artifacts that cause real-world impact, benefiting finish users past the academic community. Continue reading “Research Area: HCI EECS at UC Berkeley”
Multiple time-scales and the developmental dynamics of social systems
Jessica C. Flack
1Center for Complexity and Collective Computation, Wisconsin Institute for Discovery, Madison, WI 53715, USA
2Santa Fe Institute, Santa Fe, NM 87501, USA
Continue reading “Multiple time-scales and the developmental dynamics of social systems”
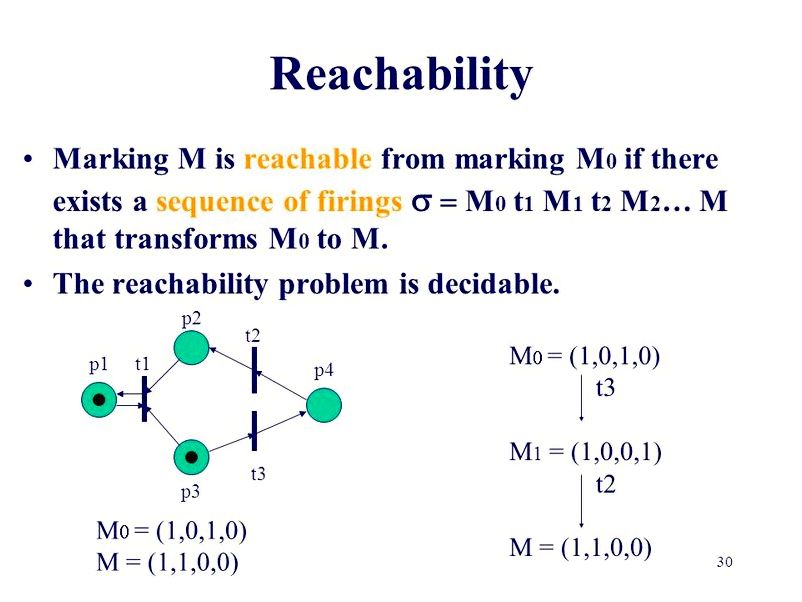
Around the Decidability of Reachability in Continuous Time Straight line Time-Invariant Systems – NASA/ADS
Abstract
We think about the decidability of condition-to-condition reachability in straight line time-invariant control systems over continuous time. We analyse this issue with regards to the allowable control sets, that are assumed is the image within straight line map from the unit hypercube. This naturally models bounded (sometimes known as saturated) controls. Decidability from the form of the reachability condition in which control sets are affine subspaces of $mathbb^n$ is really a fundamental lead to control theory. Our first outcome is decidability in 2 dimensions ($n=2$) when the matrix $A$ satisfies some spectral conditions, and conditional decidablility generally. When the transformation matrix $A$ is diagonal with rational records (or rational multiples of the identical algebraic number) then your reachability issue is decidable. When the transformation matrix $A$ has only real eigenvalues, the reachability issue is conditionally decidable. Time-bounded reachability issue is conditionally decidable, and unconditionally decidable in 2 dimensions. A lot of our decidability answers are conditional for the reason that they depend around the decidability of certain mathematical theories, namely the idea from the reals with exponential ($mathfrak_$) with bounded sine ($mathfrak_crime$). We get yourself a hardness result for any mild generalization from the problem in which the target is straightforward set (hypercube of dimension $n-1$ or hyperplane) rather of the point, and also the control set is really a convex bounded polytope. Within this situation, we reveal that the issue is a minimum of as hard because the emph or even the emph.